Thermodynamic uncertainty relation
The thermodynamic uncertainty relation reveals that higher precision, i. e. narrower distributions of fluctuating quantities, can only be achieved if the dissipation rate increases. In other words: precision always comes at a price.
This result is not merely an intriguing property of general Markov networks, it has far-reaching consequences in many systems reaching from biochemical networks to nanomachines like molecular motors and heat engines.
Related Publications
- Thermodynamic Uncertainty Relation for Biomolecular Processes, A. C. Barato, and U. Seifert, Phys. Rev. Lett. 114, 158101 (2015), Abstract, Download
- Universal Trade-Off between Power, Efficiency, and Constancy in Steady-State Heat Engines, P. Pietzonka, and U. Seifert, Phys. Rev. Lett. 120, 190602 (2018), Abstract, Download
- Finite-time generalization of the thermodynamic uncertainty relation, P. Pietzonka, F. Ritort, and U. Seifert, Phys. Rev. E 96, 012101 (2017), Abstract, Download
- Universal bound on the efficiency of molecular motors, P. Pietzonka, A. C. Barato, and U. Seifert, J. Stat. Mech. 2016, 124004 (2016), Abstract, Download
- Universal bounds on current fluctuations, P. Pietzonka, A. C. Barato, and U. Seifert, Phys. Rev. E 93, 052145 (2016), Abstract, Download
Field-theoretic thermodynamic uncertainty relation
One of the intriguing properties of the TUR is its broad, general formulation which is possible without implying a particular underlying physical model. Unfortunately, formulating the TUR across different physical models does not mean we have proved it. Thus, transferring the concepts of the TUR from its original setting to novel frameworks serves a twofold purpose: While we gain a better understanding of the general validity of the TUR in a particular model, the model itself also enjoys the new thermodynamic perspectives motivated by studying the TUR.
An accurate physical description of, say, flow patterns of a fluid or profiles of growing interfaces has to account for random fluctuations at different points in space and time and their mutual correlations. Mathematically, the appropriate model is given by stochastic partial differential equations or, in other words, field-theoretic Langevin equations. We have successfully developed a framework in this setting in which the TUR can be studied both analytically and numerically.
Related Publications
- Numerical study of the thermodynamic uncertainty relation for the KPZ-equation, O. Niggemann and U. Seifert, J. Stat. Phys. 182, 25, 2021, Abstract, Download
- The two scaling regimes of the thermodynamic uncertainty relation for the KPZ-equation, O. Niggemann and U. Seifert, J. Stat. Phys. 186, 3, 2022, Abstract, Download
Time-dependent driving
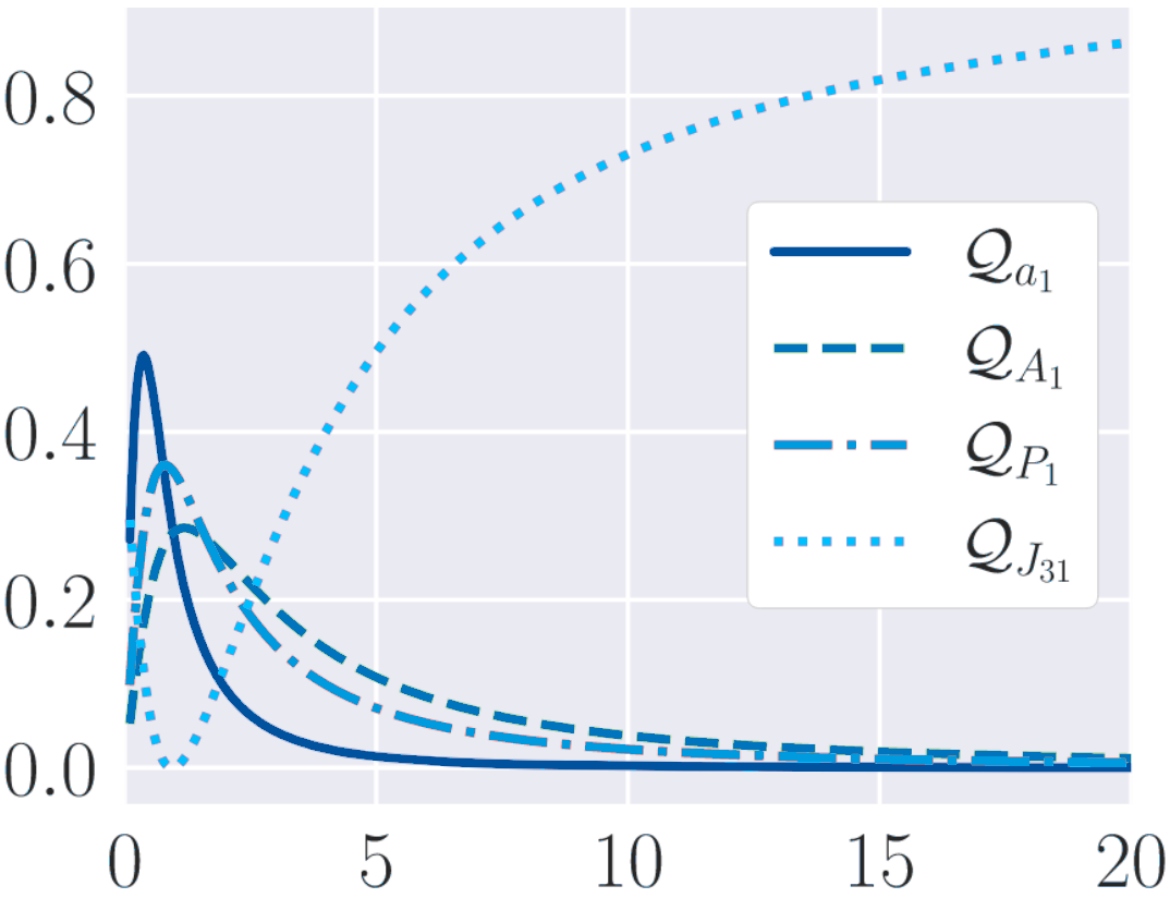
While the concepts which are incorporated in the TUR are of a very general nature, the TUR in its original form applies only to the very limited setting of non-equilibrium steady states. We generalize the TUR to Markov networks and Langevin systems under arbitrary external driving, which in particular includes relaxation processes and periodic driving.
Since this generalization is formulated in terms of operationally accessible quantities, the result is not only of theoretical interest, but also allows estimation of the entropy production in real-world scenarios if some observables can be measured experimentally.
Related Publications
- Thermodynamic Uncertainty Relation for Time-Dependent Driving, T. Koyuk and U. Seifert, PRL 125, 260604 (2020), Abstract, Download
- Quality of the thermodynamic uncertainty relation for fast and slow driving, T. Koyuk and U. Seifert, J. Phys. A: Math. Theor. 54, 414005 (2021), Abstract Download
- Operationally Accessible Bounds on Fluctuations and Entropy Production in Periodically Driven Systems, T. Koyuk and U. Seifert, Phys. Rev. Lett. 122, 230601 (2019), Abstract Download
- A generalization of the thermodynamic uncertainty relation to periodically driven systems, T. Koyuk, U. Seifert, and P. Pietzonka, J. Phys. A: Math. Theor. 52, 02LT02 (2019), Abstract Download